Estimation of the Latitude, the Gnomon’s Length and Position About Sinbeop-Jipyeong-Ilgu in the Late of Joseon Dynasty

To obtain detailed information on the lines and features of the horizontal sundial, a three-dimensional scanner was used with the optical triangulation method. The optical triangulation method can be classified into a fringe projection type and an optical triangulation laser type. The former obtains the information through illuminating a light source onto the object, and the latter acquires the information on an object by shooting a laser (Choi & Ko 2017).

In the case of the T840 horizontal sundial, 3D scan measures were conducted in 2015 using the ATOS Compact Scan 5M (GOM, Germany). This is based on the fringe projection-type phase shift pattern method. The data precision of the 3D scanner was 0.001 mm ~ 0.02 mm, and the resolution of the camera was 2,448 × 2,050 pixels. The projection method was the phase shift pattern method, and the light source was blue light-emitting diode (LED). Based on the aforementioned settings, highresolution 3D data could be obtained. The fringe projection-type 3D scanner (Fig. 1(a)) consisted of a light source, an illuminant lens, and a charge coupled device (CCD) (Lee et al. 2012).

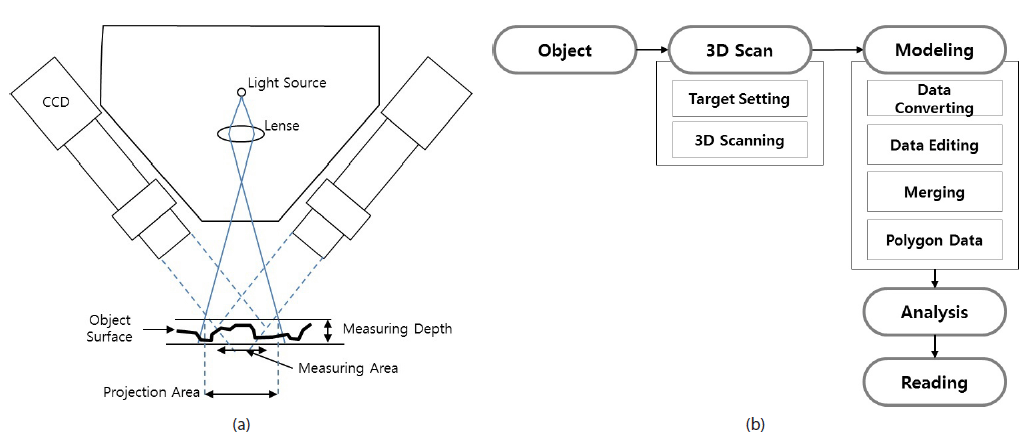
In the fringe projection measurement, a dispersive light through the lens illuminates an object, and the CCD camera detects the illuminated light reflected from the object. At the same time, the depth pattern of an object surface is analyzed from the detected light, and the three-dimensional shape of the object surface is digitized. Fig. 1(b) shows the 3D scanning and analysis process for the horizontal sundial used in the present study. The work process consists of the 3D scanning stage and modeling stage for the horizontal sundial. In the scanning stage, the measurement area of the object is determined at first and then scanned at the optimum distance of the scanner for that area (Choi & Ko 2017). The raw scan data is converted into an editable data format. The data is optimized to 3D points through a noise removing process and a hole filling process. The 3D point data of each detection area is optimized and merged to finally become polygon data (Choi & Ko 2017). Through these modeling stages the polygon data contains the depth information of the sundial’s surface where the hour-lines and solar-term curves are engraved. For the colors according to the depth ranges, the deeper the part is, the darker it gets, and the shallower the part is, the brighter it gets. When the polygon image is assigned black intensity depending on the depth, it becomes a complanation image such as the one shown in Fig. 2.

Fig. 2 shows the complanation image of T840 (hereafter ‘T840-plate’). As in the original form of the T840-plate, the center line of all hour-lines (i.e., 12^h-line) are rotated by about 17.242° in the clockwise direction relative to the vertical line of the black stone. This sundial has a total of 13 hour-lines (every hour from 6:00 to 18:00), and a total of 11 solar-term-lines that represent the 24 solar terms. However, there are not 13 solar-term-lines. A curve for Bearded grain (芒種) and Great heat (大暑) are used as the summer solstice line together, and in the same manner a curve for Heavy snow (大雪) and Slight cold (小寒) are used as the winter solstice line. In addition, there is a horn-shaped groove with a length of 43.1 mm below the inscription that reads ‘新法 地平日晷’ indicating a Western-style horizontal sundial. An inscription that states that the latitude of this sundial is 37° 39´ is engraved on the southern lateral face of this horizontal sundial (i.e., the upper side of Fig. 2).

The coordinate system of the horizontal sundial can be conveniently specified as shown in Fig. 2. The origin of this coordinate system is identical to the convergent point obtained by extending the hour-lines of a horizontal sundial (Lee 1982). It is generally set up so that the west in the horizontal plane is determined as the X-axis, and the south as the Y-axis. Fig. 3 shows the diagram for the gnomon’s projection for a horizontal sundial. Point A is the position of the gnomon, and point C is the position where the shadow of the gnomon’s tip is cast, that lies on a line of the vernal and autumnal equinoxes. The direction from the origin toward the gnomon’s tip is the North celestial pole, and thus the angle between OB and a horizon is identical to the latitude of its sundial. When the length of the gnomon is H and the declination of the sun is δ, D is a shadow position of the gnomon’s tip on the 12^h-line and AD is N.

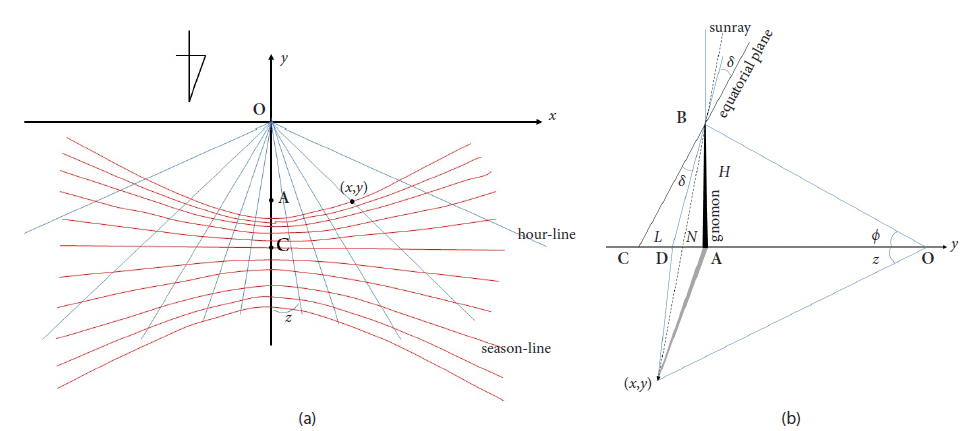
Fig. 4 shows the convergent point of each hour-line in the T840-plate. When the convergent point was magnified, it is not only one point, but is a lot of intersection points. Owing to an eccentric error of hour lines, the intersection points between the noon line (12^h-line) and the other hour-lines are dispersed from a virtual convergent point. In Fig. 4(b), each intersection point was expressed as symbols (P1, P2, P3, …, M1, M2, M3…, P6 = M6). The center point of the east-west line (AA) is also one of the intersection points. If these intersection points are eligible to be a convergent point, then they are assumed as the origins of the sundial coordinate system. These origins have characteristics similar to the convergent point’s samples which can be analyzed statistically. In other words, when each intersection point lies as the origin of the sundial coordinate, the latitude of the sundial and the length of the gnomon can be inversely estimated from the hour-lines and solar-term-lines at each origin.

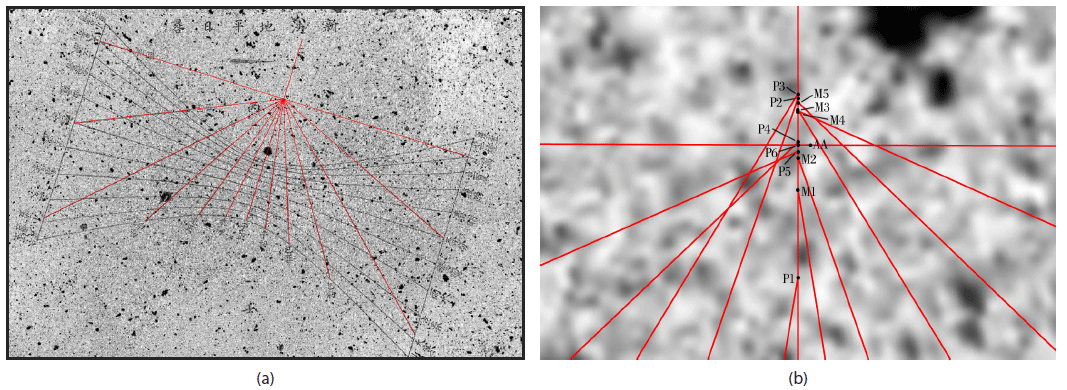
The inscription of T840 specifies the latitude of Hanyang (Seoul) installed in this sundial. For each origin shown in Fig. 4(b), the latitude of the sundial can be estimated based on the angle between the 12^h-line and the hour-line (z) shown in Fig. 4(a) (Lee 1982;Rohr 1996).

where ϕ is the calculated latitude of the sundial, HA is the hour angle, and z is the angle between the 12^h-line and the hour-line. The dummy index i represents the 12 origins, and j represents the 12 hour angles. In Eq. (1), the mean latitude of the sundial for each origin can be calculated as ${\varphi }_i={\displaystyle \sum }_j{\varphi }_{ij}$.

On the other hand, the intersection point between the 12^h-line and a solar-term-line (0, y) corresponds to a point D_j (j = 1, 2, 3, …) in Fig. 3(b). The latitude of the sundial can also be calculated from two solar-term-lines with the same declination of the sun (Lee 1985).

where y_ik is the y value of each solar-term point on the 12^h-line measured from T840-plate. The dummy index i represents the 12 origins, and j represents the 6 solar-term-lines (declination of the sun).

Based on the mean latitude of the sundial obtained by Eq. (1) and the intersection points (x, y) of each solar-termline and the hour-line, the length of the gnomon (H) can be calculated as follows.

where the dummy index i represents the 12 origins, j represents the 12 hour angles, and k represents the 6 solar-term-lines. In Eq. (3), the mean gnomon length for each solar-term-line $\left(H_{ik}={\displaystyle \sum }_j{H}_{ijk}\right)$ or the mean gnomon length for each hour-line $\left(H_{ik}={\displaystyle \sum }_k{H}_{ijk}\right)$ can be calculated for each origin. Also, the mean gnomon length for all origins is $H_i={\displaystyle \sum }_{j,\hspace{0.17em}k}{H}_{ijk}$.

With the y_ik in Eq. (2) and the values in Eq. (3), the distance from each D_k on the 12^h-line to the gnomon (A), $N_k=\overline{A{D}_k}$ can be calculated as follows.

The mean distance from each solar-term point on the 12^h-line to the gnomon can be calculated as $N_k={\displaystyle \sum }_i{N}_{ik}$. In Fig. 3(b), $\overline{O_{i}A}=\overline{O_i{D}_k}-N_k$.

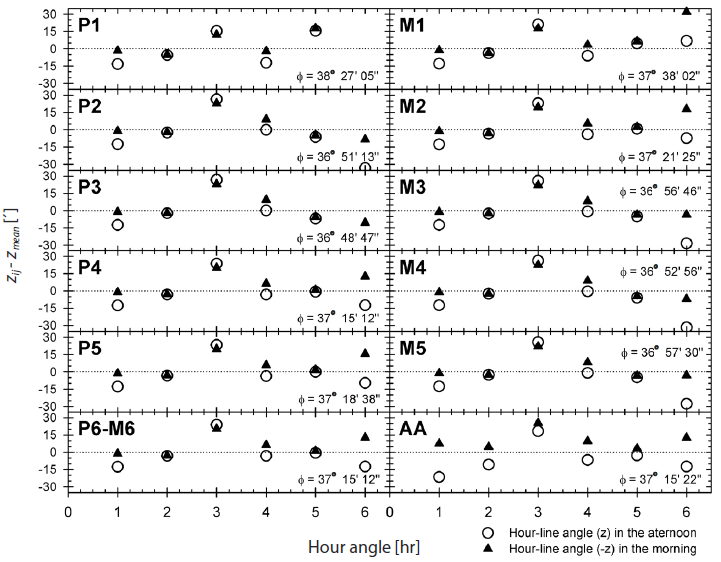
The ϕ_ij calculated by Eq. (1) is analyzed statistically for a given origin. As shown in Fig. 5, the ϕ_ij for the origin AA could be indirectly compared with the actual trajectory of the mean latitude (ϕ_AA). The abscissa is the hour angle, and the ordinate is z. For each of the given origins of i, the ϕ_ij calculated for each hour angle was different from the mean latitude (ϕ_i), and they were consistent within a maximum of 42´. In this regard, the difference between z_mean and z_ij was several 10´, where z_mean is an hour-line’s angle calculated by mean latitude ϕ_ij for a j’s hour-line and the z_ij is j’s hour-line’s angle measured from T840-plate. Regarding the origin of other i-s , the results were also similar to that shown in Fig. 5.

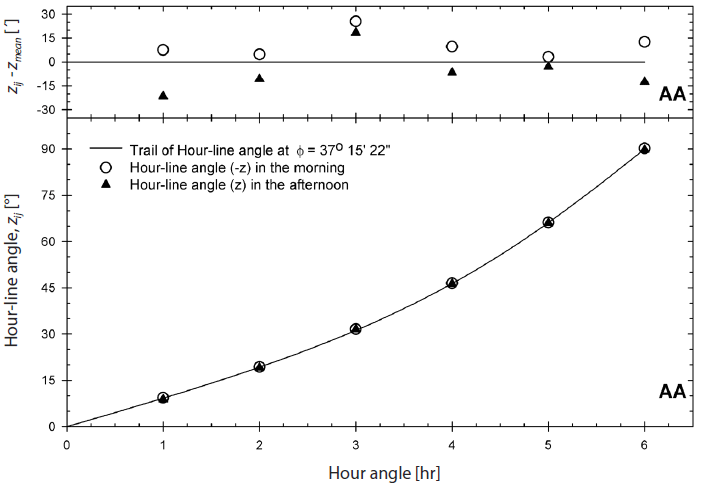
For every origin of Fig. 4(b), the mean latitude and z_ij-z_mean of a j’s hour angle is shown in Fig. 6. The mean of each origin’s mean latitude is 37° 15´ ± 26´, and this value embraces the latitude of T840 (37° 39´). However, this mean for all origins showed a difference of about 24´ compared to the actual value of the inscription.

The length of the gnomon for each origin can be estimated by Eq. (3). This value is calculated using two variables: hour angle and the declination of the sun. Accordingly, for the statistical results, the length of the gnomon (H) can also be analyzed for each hour-line and for each solar-term-line, respectively. Let H_i be the mean gnomon length of i’s origin. Let H_ij be the mean gnomon length of i’s origin and j’s hourline. Let H_ik also be the mean gnomon length of i’s origin and k’s solar-term-line. When the difference between H_i and H_ij was defined as Δ H_ij (= H_ij- H_i), the Δ H_ij is presented in Fig. 7. When the difference between H_i and H_ik is defined as Δ H_ik (= H_ik- H_i), the Δ H_ik is presented in Fig. 8. The abscissa is the hour angle, and the ordinate is the Δ H_ij in Fig. 7. The abscissa is the solar-term-line, and the ordinate is the H_ik in Fig. 8. The acronym of 24 solar terms is derived from the designation of them by Kim et al. (2017).

In Figs. 7 and 8, the Δ H_ij and Δ H_k showed slightly different distribution patterns relative to the H_i. In general, H_ij is more distributed than H_k relative to H_i. On the other hand, the standard deviation of each H_ij was smaller than that of each H_k. For example, in the case of Point AA, the mean of Δ H_ij was 0.3 mm, and that of Δ H_k was 0.2 mm. On the other hand, the mean of the standard deviations of H_ij was 0.4 mm, and that of H_k was 0.6 mm. The mean of the gnomon lengths by Eq. (3) for i’s origin ( H_i) was 43.70 ± 0.66 mm. On T840-plate (Fig. 2), the horn-shaped groove (or pin mark) below the title’s inscription reads 43.1 mm long. Although there is an error up to approximately 1 mm, the horn-shaped groove represents the length of the gnomon. It is possible that the gnomon of T840 sundial used a vertical stylus as shown in Fig. 3(b), rather than a triangular gnomon whose side is inclined from the horizontal plane by as much as the latitude of sundial (Lee 1985).

T840-plate only has hour-lines and solar-term-lines without a gnomon. Each solar-term point on the 12^h-line can estimate the position of the gnomon using Eq. (4). Let N_k be the mean distance from the gnomon to each solar-term point on the 12^h-line. The distance from the winter solstice point to each solar-term point on the 12^h-line (D_kD₁₁) can be measured from T840-plate. It can be compared with N_k + D_iD₁₁ as summarized in Table 1. The mean value of N_k + D_iD₁₁ is 76.29 ± 0.44 mm.

The $\overline{A{D}_{11}}$ measured from T840-plate was 76.0 mm. Therefore, the statistical position of the gnomon obtained by Eq. (4) is consistent with the actual position of the gnomon on the T840 sundial.