Thick Accretion Disk and Its Super Eddington Luminosity around a Spinning Black Hole

To understand the dynamics around black holes, it is essential to employ the general theory of relativity. When dealing with a number of objects, rigorous general relativistic treatment demands huge computing power and is therefore impractical. Hence, in the present work, we employ the pseudo-Newtonian potential, which involves relativistic effects on the Newtonian gravitational potential. For the case of non-spinning black holes, the pseudo- Newtonian potential is known as the Paczynsky–Witta potential, as given by Eq. (1):

In the case of spinning black holes, the pseudo-Newtonian potential for a spinning uncharged Schwarzschild black hole, Kerr black hole, charged Reissner–Nordstrom black hole, and Kerr–Newman black hole, is known as the Mukhopadhyay potential (Mukhopadhyay 2002), as given by Eq. (2):

where

In this equation, a = 0 or a = 1, where a = 0 corresponds to the Paczynsky–Witta potential.

Owing to the frictional force resulting from viscosity, the differential rotation of layers in the inner portion of the accretion disk produces outward radiation. Consequently, the gas and dust particles falling into a black hole experience outward radiation, which resists the gravitational pull of the black hole, leading to swelling of the accretion disk in the inner region. As shown in Fig. 1, this bulged portion differs substantially from the rest of the accretion disk, which is thin. According to Paczynsky and Witta, the pseudo-Newtonian potential for a Schwarzschild black hole is given by $V\left(r\right)=-\frac{GM}{r-r_g}$, where r_g = 2GM, assuming that the thickness of the disk is negligible compared to the radius of the disk. This pseudo-Newtonian potential can be safely approximated using Eq. (3):

To make our computation tractable, we introduce $R\equiv {\left(r^2+z^2\right)}^{1/2}$, where the effective potential involving the presence of centrifugal force is given by Eq. (4):

From this, we derive the gravitational acceleration (force per unit mass) as $\overrightarrow{g_{eff}}=-\nabla V_{eff}$. We now assume that the thick portion of the accretion disk is in the equilibrium state to maintain its shape. This assumption then demands that the surface of the accretion disk be on the equipotential plane, which is orthogonal to the direction of gravitational acceleration. This can be represented by the following equation:

From Eq. (5), we obtain Eq. (6).

where $R\equiv {\left(r^2+z^2\right)}^{1/2}$.

Using Eq. (6), Eq. (5) can be rewritten as follows:

To solve this differential equation, Eq. (7) is represented in a closed form.

where ω is the angular momentum per unit mass (specific angular momentum), and Eq. (8) represents the thickness of the bulging part of the accretion disk. For this calculation, caution should be exercised as ω may not denote the angular frequency for Keplerian motion, unlike the ordinary thin accretion disk. Thus, ω may deviate from the simple Keplerian angular velocity. Notably, until this point, we have considered the case of non-rotating Schwarzschild black holes, and our interest is in the case of rotating Kerr black holes. The rotating Kerr black hole adaptation of the pseudo- Newtonian potential was developed by Mukhopadhyay (2002). Therefore, in the context of the Mukopadhyay pseudo-Newtonian potential, we have

By substituting these two equations into Eq. (4), we obtain Eq. (10):

Now, we are able to describe the thick portion of an accretion disk around a black hole.

According to the Paczynsky–Witta model, in principle, the power per unit area, F_rad, is given as follows:

where g_eff denotes the acceleration, c the speed of light, and κ denotes the opacity per unit mass in the CGS (Gaussian) unit. Next, for the integration to compute the luminosity of the thick portion of an accretion disk, the surface element (dσ) is given by Eq. (12):

In the cylindrical coordinate system,

where $g_{eff}=\left|-\nabla V_{eff}\right|$, the vertical acceleration is gz = ∂V₀/∂z, and g_eff cosθ = gz. In addition, using the expressions for F_eff and cosθ, F_rad is given by Eq. (14):

Thus, the luminosity of the thick portion of an accretion disk can be represented as follows:

Finally, by substituting dz/dr and ∂V₀/∂z into this equation, we obtain,

Here, L_EDD = 4πcGM/κ = 1.3 × 10³⁸ (M/M⊙)erg/s is the Eddington luminosity, which is the critical value for the luminosity that an astrophysical object with mass M can radiate. Thus far, we have considered the case of a nonrotating Schwarzschild black hole. Similarly, we can derive the luminosity for a rotating Kerr black hole, as shown below.

where I_k can be greater than one.

The luminosity of the accretion disk is given by Eq. (17). The relations amount, X, V₀ and r are determined by the accretion rate, $\dot{M}$, according to the Shakura-Sunyaev model (Shakura & Sunyaev 1967), given by $\dot{M}\simeq \frac{\Delta }{d}{M}_{Edd}$. In general, when the disk is very thin ( Δ ≪ d ), the accretion rate is considerably lower than the Eddington accretion rate. In the present work, we study the thick portion of the disk, where the accretion rate could reach Eddington’s critical value. Notably, although an astrophysical object with super Eddington luminosity will blow up and is very unstable, when the accretion flow falls into the central black hole and the disk itself is very soft and flexible, it sustains its structure even at the super Eddington luminosity.

Therefore, we suggest that the extraordinary luminosity of a discovered quasar might be attributable to the “thick” and narrow inner part of the accretion disk surrounding the supermassive black hole, which excluding strong lensing, is the power engine for bright quasars.

First, we reproduced the results of the work by Paczynsky– Witta to study the vertical structure of a thin accretion disk in the absence of rotation. The vertical structure has already been characterized in their work, as in Eq. (18).

where l denotes the specific angular momentum, which can be written as $\frac{r^{3/2}}{r_0-1}=A_{\beta }{\left(r-r_0\right)}^B$ for numerical analysis. Employing Mathematica, we can plot this specific angular momentum, as shown in Fig. 2, even though the values for r are the same as in the work of Paczynsky–Witta.

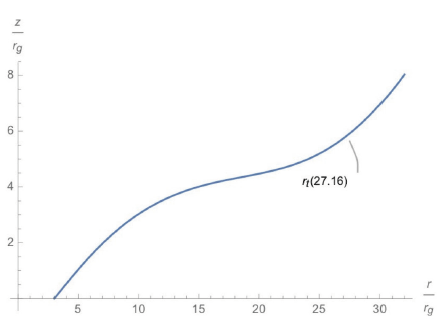
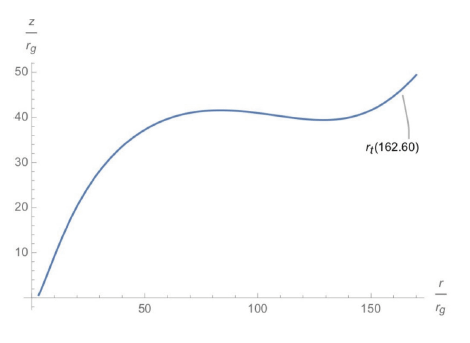
The plots above are essentially the same as those in the work of Pazcynsky and Witta. In Figs. 2–4, r_t denotes the transition radius, which specifies the boundary that separates the thin and the thick accretion disk, with $l\left(r_t\right)=\frac{r^{3/2}}{r_0-1}=A_{\beta }{\left(r-r_0\right)}^{\text{B}}$. Close inspection reveals that the closer the starting point (r₀) is to the compact object, the thicker the accretion disk becomes, and the more the disk spreads out. This allows us to speculate the appearance of accretion disk and ultimately to compare it with the theoretical computations.

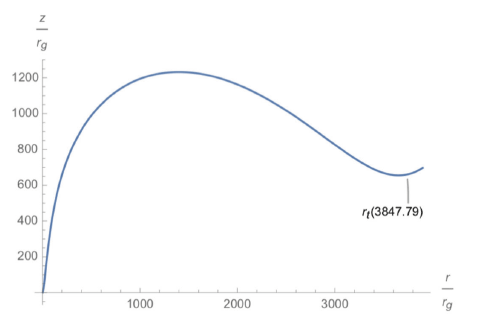
The pseudo-Newtonian potential for a spinning object is considerably more complex than that of a non-spinning object. Therefore, to investigate the vertical structure of a thick accretion disk, we adopted a numerical integration. As a result, Fig. 5–Fig. 7 ware obtained. Upon plotting the thick portion of the spinning black hole case, the shape of the disk evidently becomes steeper, particularly for the case where r₀ = 3r_g, where the disk becomes thinner but wider. That is, it is smaller vertically but larger horizontally. We conjecture that this can be attributed to the large centrifugal force of a black hole or a neutron star.