1 INTRODUCTION
Barium stars were first defined as objects with enhanced lines of barium in their spectra. Later, these stars were determined to be binary systems with a red giant primary component and a white dwarf secondary one. The white dwarf component was a red giant during the previous evolutionary stage. During the transition to white dwarf, a significant mass fraction of the former red giant was lost, and part of this material contaminated the atmosphere of the presentday red giant by s-process-enriched matter. The radiative atmospheres of red giants can conserve the anomalies of chemical composition for as long as several million years (Proffitt & Michaud 1989).
Another source of chemical anomalies in the atmospheres of main sequence and red giant stars can be the accretion of interstellar hydrogen. Discovered by Greenstein (1949), the interaction of protons with atoms of the stellar atmosphere, namely charge-exchange reactions, can be important for chemical elements with second ionization potentials close to 13.6 eV—the ionization potential of the hydrogen atom. The result is decreasing abundances of the above-mentioned elements. As the density of interstellar medium is relatively low, this type of accretion can be important only for long timescales. This process can explain the braking of rotation of magnetic peculiar stars and the origin of low energy (< 20 MeV/nucleon) cosmic rays in the Galaxy (Havnes 1970).
Later, Böhm-Vitense (2006) found that the accretion of helium atoms produces a similar effect, though near the ionization potential of helium—24.6 eV. Note that a detailed theory of this phenomenon was not developed, observed only in the peculiar stars of the upper main sequence. The observational result is the relative underabundance of chemical elements with second ionization potentials close to 13.6 and 24.6 eV in the atmospheres of stars with radiative energy transfer. To prove or reject this hypothesis, it is necessary to investigate the abundance patterns for different types of stars and find what the dependencies of relative abundances on the second ionization potentials are. It is the necessary step for constructing a theory of this type of accretion.
Kang et al. (2012, 2013) and Jeong et al. (2017) found the dependencies of relative abundances vs. the second ionization potentials in the atmospheres of the binary components of ZZ Boo, LX Per, and RR Lyn—stars with effective temperatures ranging from 5,200 to 8,000 K—and concluded that hydrogen accretion can change elemental abundances, even in stellar atmospheres with primarily convective energy transfer. Yushchenko et al. (2015) investigated the signs of hydrogen and helium accretion from the interstellar medium in atmospheres of more than two hundred stars with effective temperatures ranging from 5,900 to 15,200 K. Several results from their work will be used in our investigation. Yushchenko et al. (2017a, b) show the existence of correlations between the relative abundances and second ionization potentials in the atmospheres of two red supergiant stars in the Magellanic Clouds.
In this paper, we are going to search for signs of hydrogen accretion from the interstellar medium on atmospheres of barium stars. The matter transfer from a highly evolved component in the stellar binary system containing a barium star component was investigated before, see Husti et al. (2009) as an example. It is interesting to compare the results for two different accretion processes. The abundances of s-process elements, particularly lanthanides, strontium, yttrium, and zirconium, are strongly influenced by the accretion from a highly evolved component. Fortunately, the second ionization potentials of these elements are less than 13.6 eV, and their abundances should not be distorted by hydrogen accretion from the interstellar medium. The second ionization potentials of magnesium, silicon, and iron peak elements exceed 13.6 eV, and the relative abundances of these elements are expected to be unchanged during the transition of a binary companion from red giant to white dwarf. As can be concluded from the Yushchenko et al. (2015) results, the abundances of magnesium, silicon, and iron group elements can be changed by hydrogen accretion from the interstellar medium.
That is why the investigation of both types of accretion in barium-type stars can give valuable results. Hereafter, we use one of the most detailed chemical compositions in this star type—the composition of the mild barium star ζ Cyg (Yushchenko et al. 2004)—to show the existence of both types of accretion in its abundance pattern. Then, we select the homogeneous set of barium-type stars published by de Castro et al. (2016) to search for possible chemical anomalies produced by both accretion scenarios.
2 THE SIGNS OF ACCRETION IN Ζ CYG
The chemical composition of one of the brightest mild barium stars, ζ Cyg (HD202109), was investigated by Yushchenko et al. (2004). The abundances of 51 chemical elements were found, and hereafter, we will use this abundance pattern to search for possible signs of hydrogen accretion from the interstellar environment. Greenstein (1949) found a deficiency of relative abundances for elements with second ionization potentials close to 13.6 eV. Yushchenko et al. (2015) confirmed this result and found more observational details.
For upper main sequence stars with effective temperatures higher than approximately 7,000 K, the positive correlations between relative abundances and second ionization potentials are observed in three energy intervals, namely less than 12.5 eV, 12.5–20 eV, and higher than 20 eV. In the second of these intervals (12.5–20 eV), correlation becomes negative for stars with lower effective temperatures, namely in the effective temperature range of 5,900 K to 7,000 K. Note that Yushchenko et al. (2015) found a decrease in the abovementioned correlation for main sequence stars, in which stars with primarily radiative energy transfer in its atmospheres transition to those with primarily convective energy transfer at the effective temperatures of 6,500–7,000 K.
Fig. 1 shows the relative abundances of chemical elements in the atmosphere of giant star ζ Cyg as a function of the second ionization potentials of these elements. The picture is similar to the first panel of Fig. 6 in Yushchenko et al. (2015), where the same correlation is shown for ρ Pup—the star with effective temperature Teff = 6,890 K and the logarithm of the surface gravity g = 3.28. Note that the effective temperature and the logarithm of the surface gravity of ζ Cyg are equal to Teff = 5,050 K and log(g) = 2.8, respectively (Yushchenko et al. 2004). The star is a typical red giant, and the energy transfer in its atmosphere as well as in the case of ρ Pup is mainly radiative. Fig. 6 in Yushchenko et al. (2015) was explained as a possible superposition of hydrogen and helium accretion from the interstellar medium and other effects in the atmosphere of ρ Pup, for example, radiative diffusion. It is natural to use the same explanation for ζ Cyg.
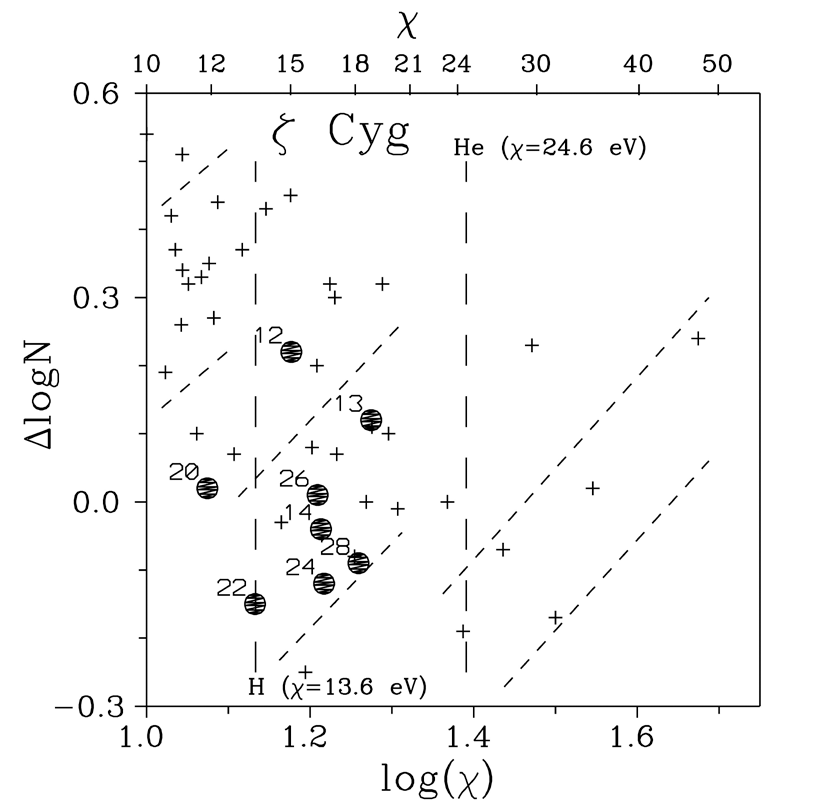
The inclined dashed lines in Fig. 1 outline the regions where the positive correlations between relative abundances and second ionization potentials are observed. Most of the elements are located in these regions, and for the rest of the elements, other effects including the influence of accretion from an evolved binary component are more significant than hydrogen and helium accretion from the interstellar medium.
3 THE SIGNS OF ACCRETION IN BARIUM STARS
As shown in the previous section, the signs of accretion can be detected in the atmosphere of mild barium star ζ Cyg. As such, it is interesting to investigate other barium stars and compare the possible observational effects produced by two different accretion scenarios. We select the homogeneous observational set of barium star abundances published by de Castro et al. (2016). This paper contains the chemical composition and kinematics for 182 barium stars and candidates. The abundances of Na, Mg, Al, Si, Ca, Ti, Cr, Fe, Ni, Y, Zr, La, Ce, and Nd were found.
We restricted our analysis to thin disk stars only. The selection was made in accordance with two criteria. One considered the probability of a star being a member of the thin disk calculated by De Castro et al. (2016). Objects with above-mentioned probabilities lower than 0.70 were rejected. The second criterion considered the space velocities with respect to the Galaxy center. We calculated these velocities in accordance with the Johhson & Soderblom (1987) method and rejected stars with an absolute value of velocity component perpendicular to the plane of the Galaxy higher than 70 km·s-1. Only 129 objects satisfied both criteria. These stars were used for the subsequent analysis. The effective temperatures of selected stars range from 4,100 K to 5,400 K, with the logarithms of surface gravity ranging from 1.1 to 3.2.
We analyzed the correlation coefficients of the relative abundances of chemical elements vs. second ionization potentials of these elements. Chemical elements with atomic numbers Z < 30 and second ionization potentials in the range of 12.5 to 20 eV were selected for the calculation of the correlation coefficients. Eight chemical elements investigated by de Castro et al. (2016) satisfied these conditions, namely Mg, Al, Si, Ca, Ti, Cr, Fe, and Ni. These elements are shown by filled circles in Fig. 1.
Correlation coefficients between the relative abundances of the above-mentioned eight chemical elements and their second ionization potentials were calculated for 129 thin disk barium stars taken from de Castro et al. (2016). Hereafter, we will call them ΔlogN-χ correlations. These correlation coefficients were used to find second order correlations— the dependencies between ΔlogN-χ correlations and other characteristics of the investigated stars. Several valuable cases were found.
If the accretion of hydrogen from the interstellar medium can influence the chemical composition of barium stars, it is natural to suppose the results of this accretion are dependent on the spatial velocities of the stars. Fig. 2 shows the plot of ΔlogN-χ correlations for 129 thin disk barium stars as a function of the spatial velocities of these stars.

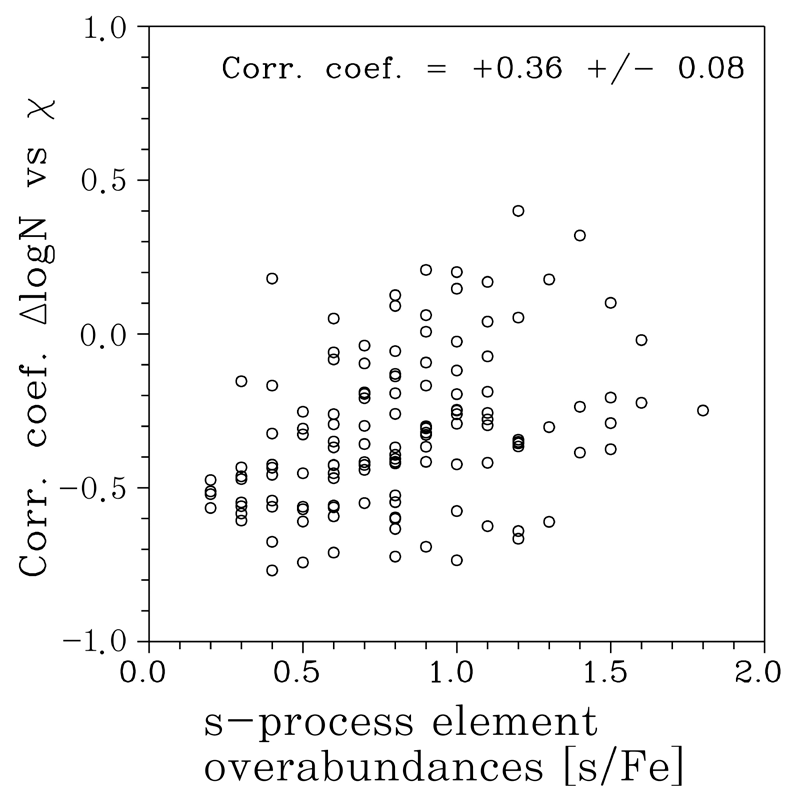
To investigate the physical interactions in some different accretion scenarios, namely the accretion from the evolved binary companion and the hydrogen accretion from the interstellar medium, it is interesting to view the plot of ΔlogN-χ correlations as a function of s-process element overabundances. This plot is shown by Fig. 3. The mean s-process element overabundances [s/Fe] are defined by de Castro et al. (2016) as the mean overabundances of Y, Zr, La, Ce, and Nd with respect to iron.
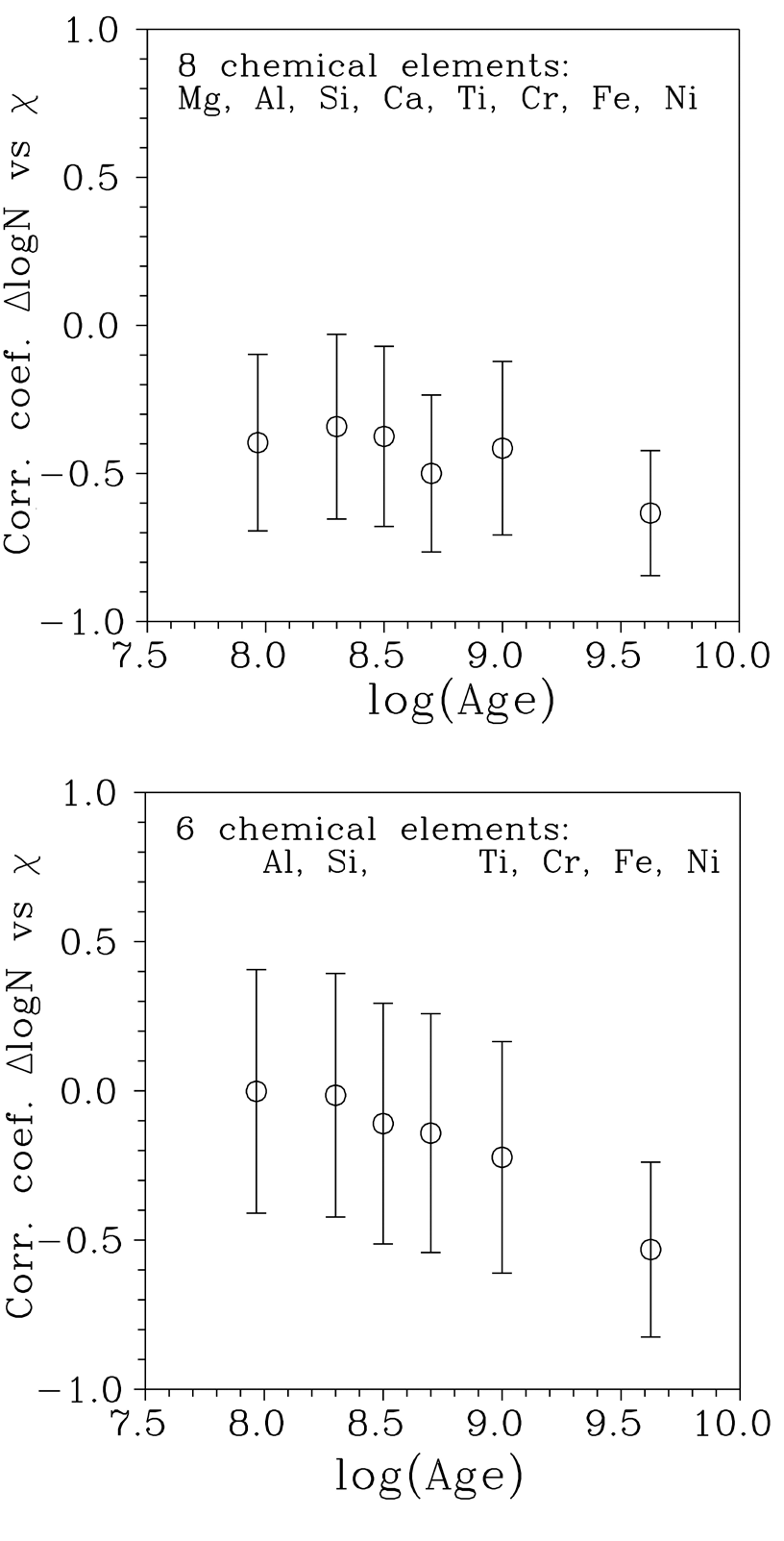
The accretion of hydrogen from the interstellar medium can be more important at long timescales. Therefore, determining the age dependence of ΔlogN-χ correlations was attempted. For this purpose, 129 stars were distributed in six groups with different ages, estimated by de Castro et al. (2016). For all these groups the mean value of ΔlogN-χ correlation was found. Note that individual correlations were found for the eight chemical elements discussed previously and also for six other chemical elements, namely Al, Si, Ti, Cr, Fe, and Ni. Table 1 and Fig. 4 show the results of these calculations. The mean correlation coefficients decrease with age. The effect is very small in the case of correlations, calculated for eight chemical elements, but it becomes noticeable in the case of six-element correlation coefficients.
4 CONCLUSIONS
As discussed in Section 2, the dependence of the relative abundances of chemical elements in the atmosphere of ζ Cyg vs. second ionization potentials of these elements is similar to the dependence in the atmosphere of ρ Pup. That is why we can conclude the possible existence of hydrogen and helium accretion from the interstellar medium in the atmosphere of ζ Cyg. Similar to the case of ρ Pup, there are many elements that do not follow the correlations described in Section 2. For these elements we can suppose the strong influence of other physical effects. Examples of these effects are the accretion from an evolved binary companion and the radiative diffusion in a stellar atmosphere.
Note that in Fig. 1, we pointed out several regions with possible correlations defined by inclined dashed lines. The correlation coefficients inside these regions are clearly positive. For example, the correlation coefficient between the relative abundances of Al, Si, Ti, Cr, Fe, and Ni and the second ionization potentials of these elements is equal to +0.66±0.23. However, if we add the relative abundances of Mg and Ca, the correlation coefficient for the eight chemical elements will be close to zero, namely +0.07±0.35. The abundances of magnesium and calcium can be changed due to other scenarios, for example, the presence of radiative diffusion (Michaud 1970).
This explains the fact that most of the 129 thin disk barium stars discussed in Section 3 exhibit zero or negative ΔlogN-χ correlation coefficients calculated for the eight chemical elements only.
Investigating a more detailed abundance pattern would be preferable, but the de Castro et al. (2016) set of barium star abundances is a homogeneous one, facilitating the search for a possible dependence between the ΔlogN-χ correlation coefficients calculated for 129 thin disk barium stars and other characteristics of these stars, namely, the spatial velocities, mean overabundances of s-process elements, and ages. As discussed by Yushchenko et al. (2015), these dependencies can be the signs of possible hydrogen and helium accretion from the interstellar medium.
Note that the exact theory of this type accretion was not constructed. At the present time, it is important to collect the possible observational evidence of this phenomenon to have more features explained in future theories. Of course, we cannot exclude the possibility that the abovedescribed observational facts can be the result of other physical scenarios. However, in any case, we have several new interesting correlations to be explained in future investigations.









