Astronomical Instruments with Two Scales Drawn on Their Common Circumference of Rings in the Joseon Dynasty

The Small Simplified Armillary Sphere and the Sun-and-Stars Time-Determining Instrument were first made in 1433 and 1437, respectively, under the reign of King Sejong of Joseon. Both two instruments have all three rings, with the scale engraved on each. The instruments can be characterized as observation instrument and clock, respectively, by extracting the corresponding function from the Simplified Armilla (Kim et al. 2012).

Mihn et al. (2015) summarized the specifications of the Small Simplified Armillary Sphere and the Sun-and Star Time-Determining Instrument in detail. The Sun-and Star Time-Determining Instrument has three concentric rings which are placed on the equator; the Celestial-circumference degree-and-fraction ring, the Sundial Hundred-interval ring, and the Star-dial Hundred-interval ring. The outermost ring is 2.00 ja (41.4 cm) in diameter and is also engraved by graduations of the Celestial-circumference degree, which is 365.25 du in a turn. The middle and the inner ring have Hundred-interval graduations with 1.84 ja (38.1 cm) and 1.68 ja (43.8 cm) in diameter respectively (Mihn et al. 2015). The scale of the Hundred-interval comes from 12 double-hours and 100 intervals per day in Chinese astronomy.

The Small Simplified Armillary Sphere also has concentric rings; two concentric rings and the other ring perpendicular to them. On the equatorial plane, the Hundred-interval ring is fixed within the equatorial ring. The former is 1.84 ja in diameter and the latter is 2.00 ja (Mihn et al. 2015). It is different from that the fixed Hundred-interval ring of the Simplified Armilla is out of its equatorial ring (Lee 1996). The declination ring of the Small Simplified Armillary Sphere is perpendicular to the equatorial plane but identical to the equatorial ring in terms of the size and the scale. The equatorial ring has the scale of 28 lunar lodges as well as Celestial-circumference degree, while the declination ring has a half of the Celestial-circumference degree scale on each of the two semicircles, which is divided by the two poles; the North pole and South pole. The analysis of the connection between a ring’s size and its scale is made only with those of the Sun-and Star Time-Determining Instrument, which is greatly similar to aspects of the Small Simplified Armillary Sphere.

There are two shapes of rings historically employed in astronomical instruments of Korea and China; one is a thin flat ring which resembles a washer like Fig. 1(a) and the other is a thin band ring like Fig. 1(b). Because the ticks for scale were drawn on the circumference of the ring (Mihn et al. 2015), a thin flat ring has at least two circumferences of an outer diameter and an inner diameter on which t scales can be marked (see the Fig. 1). In this paper, the inner, the middle, and the outer circumference of a thin flat ring are considered.

Fig. 1. The shape of the ring used in an armillary sphere. (a) A thin flat ring can create many circumferences for scale, but (b) a thin band ring has a single circumference if its thickness is not broad.

Download Original Figure

The specifications of a ring are expressed as diameter, width and thickness. In the Chinese literature, the diameter means the outer diameter, regardless of whether it is a thin flat ring or a thin band ring. The inner diameter of a thin flat ring is determined by subtracting two times the width from the outer diameter. In the same way, the inside diameter of a thin band ring is determined by subtracting two times the thickness from the outside diameter, and is negligible if that thickness is small.

An example of a thin flat ring is the three rings of the Sun-and Star Time-Determining Instrument as well as the Small Simplified Armillary Sphere. Because the diameter and width of the outermost ring are 2.00 ja and 0.08 ja, respectively (Mihn et al. 2015), it can be easily seen that 1.84 ja is the inner diameter, which is equal to the size of the next inner ring at the same time. The inner diameter of the middle ring is also identical to the (outer) diameter of the innermost ring.

An example of a thin flat ring is a Chinese armillary sphere (or Ptolemaic armillary sphere), which was mainly made by Joseon Neo-Confucians out of bamboo or other wood. A Chinese armillary sphere usually formed in two or three layers; the outermost layer to fix the polar axis, the middle layer to move objects such as the sun and the moon, and the innermost layer for viewing stars. The outermost layer is united by a meridian (double) ring, an east-west or equatorial ring and horizontal ring. The middle layer combines a solstitial colure, an equinoctial colure, an equatorial ring, an ecliptic ring and so forth. Each layer is formed a sphere as joining several points of each ring. The innermost layer is placed by a declination ring.

In pre-modern China and Korea, the scale of rings was directly drawn on the circumference by splitting the necessary ticks equally, rather than using a protractor (Mihn et al. 2015).

Here d is the diameter of a circle, a is the size of the unit scale. ∏ means the ratio of the circumference to the diameter, for which 3 was used in China but is more accurately π = 3.141592…. κ is the ratio of division which is 100 in Hundred-interval scale, and is 365.25 in Celestial-circumference degree scale.

In the past China it is actually convenient to select a ring’s diameter of which circumference can be divided into the Celestial-circumference degree or the Hundred-interval graduation. However it is not easy to use only an integer times circumference when many rings are entangled with each other or an instrument itself is miniaturized like the Sun-and-Stars Time-Determining Instrument.

When we work on the premise that $a=\raisebox{1ex}{$n$}\!\left/ \!\raisebox{-1ex}{$m$}\right.$, where n and m are all integers, Eq. (1) is changed to the following.

Here, c is the unit conversion constant if c=100, and the unit of a (or n) changes from ja to pun. (1 ja = 10 chi = 100 pun ). For example, where κ=365.25 [du], Eq. (2) means looking for d [ja] when it is n [pun] per m [du] and c=100. Table 1 shows diameters with the integer scale of unit length in Celestial-circumference degree (du) and the Hundred-interval with regard to ∏ (Mihn et al. 2015).

Table 1. Diameter with a multiple integer scale of unit length in the Celestial-circumference degree (du) and the Hundred-interval as to Π (see Mihn et al. 2015)

Download Excel Table

Entering the 18^th century, the nonius scale (橫截線) with a transversal line was introduced in Joseon. According to the Chapter ‘Armillas and Gobles (曆象)’ in the Gukjo-ryeoksang-go, the Equatorial Armillary (赤道經緯儀) and the Horizontal Sundial (地平日晷) were made by Kim Yeong (金泳) in 1789 (Lee & Moon 2004).

The Equatorial Armillary has the median ring (子午規), the equatorial ring (赤道規) and the declination ring (四遊規). The Median ring is the biggest ring and is drawn with a Babylonian 360-degree scale, which was brought from the West, rather than 365.25 du. Every degree is divided into 6 parts on a transversal line. This transversal line for scale is used on instruments made by Tycho Brahe in Europe (Brahe 1602;Chapman 1989) and then was brought into China by Catholic missionaries in the period from the late Ming to the early Qing. Jacques Rho (羅雅谷, 1593-1638) and Johann Adam Schall von Bell (湯若望, 1591-1666) in the Celiang quanyi (測量全義, Complete Definition of Measurements) had introduced this nonius scale and applied it to the 1/10 degree scale of many instruments installed on the Beijing Old Astronomical Observatory (古觀象臺) (Zhang 2000). The nonius scale of Brahe’s instruments kept declination error within about 2´ in his observations (Wesley 1978).

However, in the 15^th century, when the Small Simplified Armillary Sphere and the Sun-and Star Time-Determining Instrument were manufactured, it was not known how to divide a graduation into several parts. A right-angled triangle might have been used to divide m du into n pun. How to divide m du into n pun is shown in Fig. 2. First, draw a right-angled triangle with a 3:4:5 length ratio to match the scale of npun. Second, draw the m-1 lines parallel to another side from m-1 points every one pun of the m-pun side to the n-pun side of the right-angled triangle. Then, m parts on the n-pun side can be made equally.

Fig. 2. Dividing a scale by using the right-angled triangle with a 3:4:5 length ratio.

Download Original Figure

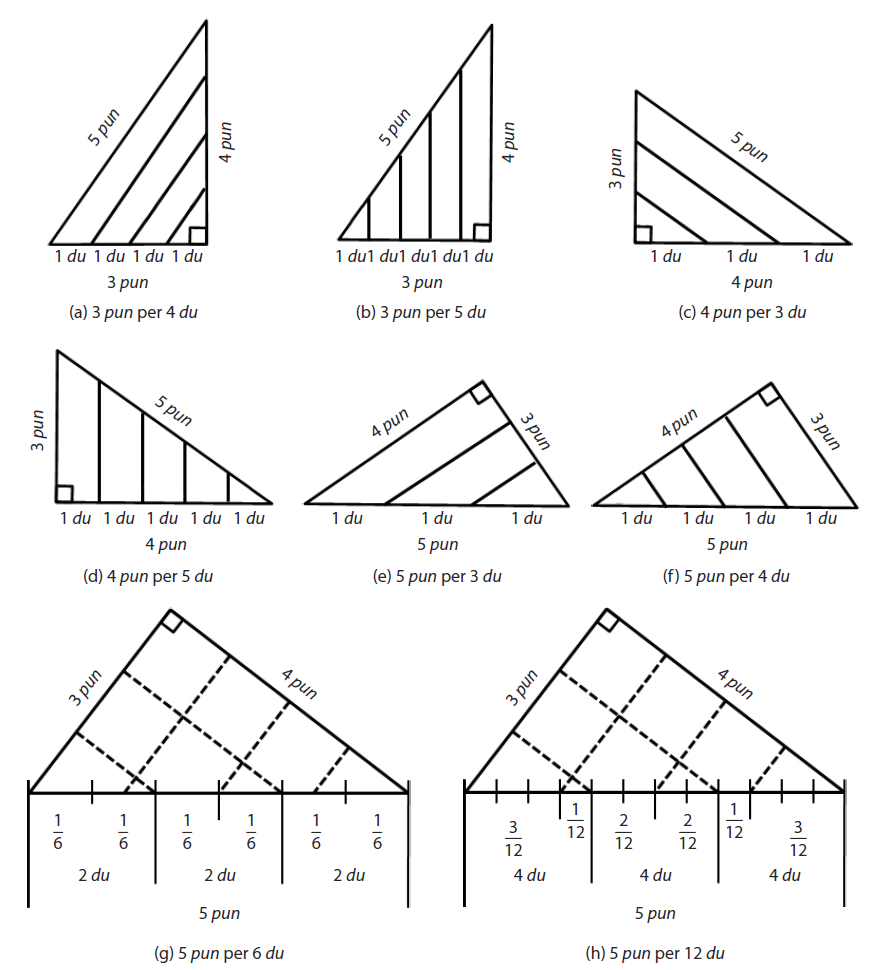
In the right-angled triangle with a 3:4:5 length ratio, 1 du can be separated into the units of 3/5 pun, 3/4 pun, 4/5 pun, 5/6 pun, 5/4 pun, 4/3 pun, and 5/3 pun. Since 1 punis 2.07 mm, if m-pun side is divided into 0.5 pun units, 1 du of n-pun side can be divided into 3/10 pun, 3/8 pun, 4/10 pun, 5/12 pun, 5/8 pun, 3/8 pun, 4/6 pun, and 5/6 pun. Table 2 shows diameters of the miniaturized rings with sub-pun scale for the Celestial-circumference degree by virtually drawing a right-angled triangle.

Table 2. Fractional division of the Celestial-Circumference degree scale (κ = 365.25) applied to the right-angled triangle

Download Excel Table

It is considered that the Sun-and-Stars Time-Determining Instrument and the Small Simplified Armillary Sphere are astronomical instruments structurally simplified by trans-forming the Simplified Armilla (Kim et al. 2012). The Simplified Armilla, which was first designed by Guo Shoujing (1231~1316), has a distinctive structure from an armillary sphere, also a traditional instrument in old China. The specifications of the Simplified Armilla, which are defined in the Astronomy Treatise of the Yuanshi (元史, History of the Yuan Dynasty), are extremely similar to its reconstruction in the Ming dynasty (Nha et al. 1994). In the Simplified Armilla, the equatorial ring is a inscribed ring about the Hundred-interval ring; with the former 6.0 ja in diameter and the latter 6.4 ja. According to the rubbing of the Simplified Armilla of the Ming dynasty (Nha et al. 1994), the Celestial-circumference scale of the equatorial ring faces the hundred interval time scale of the Hundred-interval ring on the circumference of 6.0 ja in the diameter. In other word, the Celestial-circumference scale is carved on the outer circle of the equatorial ring and the Hundred-interval scale is done on the inner circle of its own ring.

In Table 1, when ∏=3 and 1 du in the Celestial-circumfer-ence degree is 5 pun long, then the diameter of that circle is 6.09 ja. Also, when 1 interval in the Hundred-interval scale is 18 pun long, then the diameter of that circle is 6.0 ja. The two diameter values calculated from the different scale system are similar to each other and are comparable to the value reported by Nha et al. (1994). Moreover, as recorded by the Astronomy Treatise of the Yuanshi, every interval of the Hundred-interval ring was split into 36 parts and every du of the equatorial ring was divided into 10 parts in the Simplified Armilla. Dividing by 0.5 pun, every interval (1 interval = 16 pun) and every du (1 du = 5 pun) can easily make 36 parts and 10 parts, respectively. Meanwhile in Table 1, when ∏=3.14, the diameter of the Hundred-interval ring is 6.05 ja if 1 interval is 19 pun and the diameter of the equatorial ring is 5.82 ja if 1 du is 5 pun. This, while not impossible, is not as easy as dividing into 36 parts from 19 pun as 18 pun in the Hundred-interval ring. It also has a significant difference of 0.18 ja (4.6 cm) between the recorded value of the equatorial ring of Yuanshi and the calculation in Table 1.

The Sun-and-Stars Time-Determining Instrument was designed to take advantage of the Hundred-interval ring, the equatorial ring as well as the Polar-fixing ring of the Simplified Armilla. It can be used to measure the nocturnal time by adding another Hundred-interval ring. In the Sun-and-Stars Time-Determining Instrument these were called the Sundial Hundred-interval ring, the Celestial-circumference ring, and the Star-dial Hundred-interval ring, respectively (Needham et al. 1986). Unlike the Simplified Armilla, the Celestial-circumference ring is an inscribed ring about the Sundial Hundred-interval ring. As mentioned earlier, this alignment is the same as in the Small Simplified Armillary Sphere, in which the equatorial ring is also out of the Hundred-interval ring. As was described in the Sejong Sillok, every du of the Celestial-circumference ring was divided into 4 parts, and every interval of the two Hundred-interval ring was split into 6 parts.

From the Simplified Sundial, it can be considered that the Hundred-interval scale is carved on the outer circle of the Sundial Hundred-interval ring of the Sun-and-Stars Time-Determining Instrument (Lee et al. 2016). This artifact has a single ring with the Hundred-interval scale of which every interval has 6 divisions, like the description of the Sejong Sillok. This ring is 40.2 cm in outer diameter, 31.0 cm in inner diameter, and 4.52 cm wide, which can be converted to 1.94 ja, 1.05 ja, and 0.22 ja individually.

In the summary of Mihn et al. (2015), the three rings of the Sun-and-Stars Time-Determining Instrument are 2.00 ja, 1.84 ja and 1.68 ja in diameter, respectively. In Tables 1 and 2, when ∏=3 and if 3 du is 5 pun, then the diameter of that circle is 2.03 ja. If one interval is 5 pun, then the diameter of that circle is 1.67 ja. In these cases, the Hundred-interval scale can be drawn on the outer circumference of the innermost ring or on the inner circumference of the middle ring as shown in Fig. 2(g) (see Fig. 3). The Simplified Time-Determining Instrument is the same structure as the Sun-and-Stars Time-Determining Instrument (Lee et al. 2016), and the lateral side of a wheel and handle, on which three rings are located and maintained, is drawn with arbitrary lines dividing 1 du into 4 notches. If the Celestial-circumference scale can be on the outer circumference of the outermost ring, it is convenient that these lines are easily drawn and compare with the outer ring’s scales. In the scale of 5 pun per 3 du, if 1 du is separated into 4 ticks, it is 0.42 pun (0.86 mm) per part.

Fig. 3. Specifications of the three rings of the Sun-and-Stars Time-Determining Instruments, where Π is 3 or 3.14.

Download Original Figure

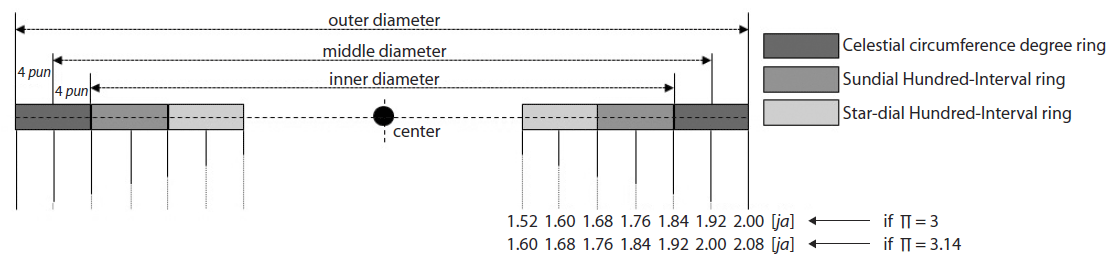
However when ∏=3, the circumference between the Celestial-circumference ring, that is the outermost ring, and the Sundial Hundred-interval ring, that is the middle ring, is not individually engraved with its own scale. In other words, the interfacial circle between the equatorial ring and the Hundred-interval ring of the Small Simplified Armillary Sphere has no scale. Differently from the example of the Simplified Armilla in Section 3.1, if ∏ is applied to 3 in two minimized Joseon instruments, then it is concluded that two kinds of scale cannot be drawn to one circumference of a circle. However, where ∏=3, Joseon astronomers could select two rings designed by each type of scale on one circle because it is 2.00 in diameter, if the interval is 6 pun. Then, the common circumference of the Celestial-circumference ring and the Sundial Hundred-interval ring is produced by a circle in the range of 2.00 ja to 2.03 ja in diameter. It is also beneficial to divide an interval into 6 parts where the interval is 6 pun.

Mihn et al. (2015) has suggested that Joseon astronomers calculated ∏ as 3 when they made astronomical instruments. When ∏=3.14 in Table 1, it is 1.91 ja in diameter if an interval is 6 pun, which is easy to divide using 6 notches, in the Sundial Hundred-interval scale. In such the ∏ in Table 2, if 3 du is 5 pun, it is 1.94 ja in diameter, which is identical to the diameter of the Hundred-interval ring of the Simplified Sundial (Song et al. 1994). Considering this Hundred-interval ring, it can be supposed that the outer circle of the Sundial Hundred-interval ring that is 1.92 ja in diameter is in contact with the inner circle of the Celestial-circumference ring. This is similarly applied to the Small Simplified Armillary Sphere. If so, as shown in Fig. 3, it is 2.08 ja in outer diameter and also 2.00 ja in middle diameter of the Celestial-circumference ring where ∏=3.14. Continuously it is 1.84 ja in the middle diameter and 1.76 ja in the inner diameter of the Sundial Hundred-interval ring, and it is 1.68 ja in the middle diameter and 1.60 ja in the inner diameter of the Star-dial Hundred-interval ring. When 3.14 is used as ∏ in Table 1, if one interval is 5 pun, then the diameter of that circle is 1.59 ja, which is close to the inner diameter of the Star-dial Hundred-interval ring. In this circle, an interval is made with 6 divisions inside by using Fig. 2(g). As the outer diameter of the Celestial-circumference ring is to be 2.08 ja, if the wheel of the same size of the Simplified Time-Determining Instrument is carved with arbitrary lines dividing 1 du into 4 notches in its lateral side, it is to be 0.45 pun (0.93 mm) per part.

Table 3 shows three ring’s sizes of two Joseon period instruments when ∏ is 3 or 3.14. In the summary of Table 3, when ∏=3.14, it is represented in the Sejong Sillok that the specification of three rings is for the middle diameter. However when ∏=3, the specification of the Sejong Sillok follows the Chinese convention by recording the ring’s size as its outer diameter. In terms of the record, it might be correct that ∏ is 3, but in terms of the Korean artifact it is correct to use 3.14 as ∏.

Table 3. Specification of the three rings of the Sun-and-Stars Time-Determining Instruments (SSTD) and the Small Simplified Armillary Sphere (SSAS)

Download Excel Table

The Celestial-circumference scale is engraved in the equatorial ring and the declination ring of the Small Simplified Armillary Sphere and is 5 pun as 3 du regardless of ∏ in Section 3.2. According to the Sejong Sillok, this equatorial ring has a scale of 28 lunar lodges as well (Needham et al. 1986). The Celestial-circumference scale is drawn in three types on the ring. One type is just the Celestial-circumference scale itself like the Celestial-circumference degree-and-fraction ring of the Sun-and-Stars Time-Determining Instrument. Another is a half of the Celestial-circumference scale (182.625 du) drawn from the south/north pole to another pole on two semicircumferences, like the declination ring. The other is the combination scale with 28 lunar lodges like the above example of the equatorial ring in the Small Simplified Armillary Sphere. The last type is identical to the equatorial ring of the Simplified Armilla (Lee et al. 1996).

The whole-degrees-in-equator (赤道宿度) of 28 lunar lodges (lunar mansions) can be found in the Chiljeongsan-naepyeon (七政算內篇, the Inner Volume of Calculation of the Motions of the Seven Celestial Determinants) (Yu et al. 1973) which was promulgated in 1443 (Lee 2007). The royal observatory of Joseon already had possessed the Shoushilijing (授時曆經) imported from Yuan and Ming in 1423 (Lee 2007) before the inscription of the Small Simplified Armillary Sphere was composed by Jeong Cho (? ~ 1434) in 1433. The whole-degrees-in-equator of 28 lunar mansions of the Chiljeongsan-naepyeon is equal to that of the Shoushi lijing. The Chiljeongsan-naepyeon said that this whole-degrees-in-equator of 28 lunar lodges is observational values obtained by using the Simplified Armilla. As mentioned above, basically a du was divided into 4 parts in the equatorial ring of the Small Simplified Armillary Sphere. Table 4 shows the whole-degrees-in-equator of 28 lunar lodges and a scale error of the equatorial ring of the Small Simplified Armillary Sphere

Table 4. The whole-degrees-in-equator of 28 lunar lodges carved in the Small Simplified Armillary Sphere

Download Excel Table

In Table 4, almost all lunar lodge’s whole-degrees-in-equator have the unit of 0.1 du except the Maiden, the Emptiness, the Bristle, the Draw-a-bow, and the Wing constellation, which have also 0.05 du in a common difference and can be easily calibrated. Moreover, the Maiden and the Emptiness constellations are successive constellations. The Draw-a-bow and the Wing constellations are the same.

In general, because the whole-degrees-in-equator of the Emptiness constellation includes a residual produced by the remaining Celestial-circumference degree out of the significant number of its scale, the origin of this scale tends to begin with the Danger constellation. That is why the start point of the Danger constellation corresponds to the rat (子) branch (the north) in the Joseon Planisphere, for instant, Cheonsang-Yeolcha-Bunya-Jido (天象列次分野之圖).

It is not possible to reconcile the subunit between the whole-degrees-in-equator of the Chiljeongsan-naepyeon and 4 parts below a du in the Small Simplified Armillary Sphere. In Table 4, each whole-degrees-in-equator of the Small Simplified Armillary is changed to an appropriate value reflecting its original whole-degree every lunar mansion. Basically, the range of 0, ¼, ½, and ¾ du is set by [-0.10, 0.10], [0.15, 0.35], [0.40, 0.06], and [0.65, 0.85] respectively. Although there is no interval of the Bristle constellation, the whole-degree of ¼ du is imposed. To eliminate the accumulated difference, the Tail and the Crossbar constellation is added ¼ du. If so, the difference between the scale of the Small Simplified Armillary Sphere and the original whole-degrees-in-equator of 28 lunar mansions corresponds to about 0.1 du in root mean square (RMS).