Burn Delay Analysis of the Lunar Orbit Insertion for Korea Pathfinder Lunar Orbiter

1 INTRODUCTION

The Republic of Korea started a lunar exploration program in 2016, and the Korea Pathfinder Lunar Orbiter (KPLO) is under development at the Korea Aerospace Research Institute (KARI). This will be the first Korean spacecraft to fly to the Moon. The total wet mass of the KPLO will be around 550 kg, with approximately 40 kg of scientific instruments; the scientific payload includes the Lunar Terrain Imager (LUTI), wide-angle Polarimetry Camera (PolCam), KPLO gammaray spectrometer (KGRS), KPLO magnetometer (KMAG), disruption tolerant network (DTN), and ShadowCam. The lunar mission orbit will be a polar circular orbit at 100 km altitude, and the KPLO will maintain an altitude of 100 ± 30 km during the nominal mission phase. The KPLO will collect the lunar scientific data during its mission period of 12 months.

The lunar orbiter will be launched from low Earth orbit, and injected into the trans-lunar trajectory to the Moon with a launch vehicle. Throughout the trans-lunar trajectory, the lunar orbiter will enter into the lunar gravitational field, and will be captured into the desired lunar orbit using several lunar orbit insertion (LOI) maneuvers. In the nominal lunar orbit, the KPLO will periodically execute maneuvers to maintain the lunar altitude boundary during the nominal operation phase. Several preliminary trajectories have been investigated to design the trans-lunar trajectory and nominal lunar orbit (Beckman 2007; Beckman & Lamb 2007; Houghton et al. 2007; Bonocoli & Fujii 2010; Bae et al. 2013a, b; Choi et al. 2013; Bae & Ju 2014; Song & Kim 2015; Lee et al. 2017). However, previous works focused on the trajectory design of the lunar orbiter, without considering the uncertainty. During operation, the KPLO will be exposed to variables, such as external disturbance, mechanical modeling error, and reaction control system (RCS) performance, which may lead to changes in the trajectory of the KPLO and the necessity for additional maneuvers to compensate for them. Previous studies have conducted dispersion analyses of the KPLO considering uncertainties in the trans-lunar injection (TLI) and LOI maneuvers. (Bae et al. 2016a, b; Song et al. 2016; Bae et al. 2017a, b).

In addition to these uncertainties, delayed burn should be considered when analyzing the trajectory of the KPLO. During the operation of the lunar orbiter, the planned LOI burn will be executed at the specific LOI burn epoch; however, the lunar orbiter may have a LOI burn delay due to hardware limitations, telemetry delays, or other factors. Because of this delayed LOI burn, the lunar orbiter will be inserted into a different captured lunar orbit at the delayed LOI burn epoch, and the orbital elements will differ from the desired states. In this situation, an additional delta-V should be required to compensate for the delayed effects on orbital parameters. In the worst case, the KPLO will be unable to insert itself into the planned lunar orbit due to a fuel shortage. Therefore, it is necessary to analyze the captured lunar orbit with respect to the LOI burn delay. In this study, a preliminary analysis of the first LOI burn delay is performed for the KPLO. The first LOI maneuver is a crucial maneuver to capture the orbit and orbit the Moon for the lunar mission. For insertion into the lunar mission orbit, several maneuvers are required, and the KPLO will use a total of four LOI maneuvers. Among these burns, the first LOI maneuver is the most difficult and important operation because the velocity of the lunar orbiter will be accelerated by the gravitational pull of the Moon. The propulsion system of the KPLO will decrease its velocity at a specific planned point, which initiates the first LOI maneuver epoch. In the worst case, the KPLO will escape from the Moon if the first LOI maneuver is executed far from the planned time. Therefore, we evaluate the burn delay of the first LOI maneuver to successfully enter the first captured lunar orbit. For this analysis, the burn delay time is given within the range from -10 to 10 min from the planned first LOI maneuver epoch. For each time delay interval, numerical simulations are performed for the sensitivity analysis.

This paper is organized as follows. In Section 2, the burn delay of the first LOI maneuver is presented. The numerical simulation and analysis in the presence of the first LOI maneuver for the lunar orbiter are described in Section 3. Finally, conclusion is presented in Section 4.

2 BURN DELAY OF THE FIRST LUNAR ORBIT INSERTION

The KPLO will be inserted into the lunar mission orbit along the trans-lunar trajectory from the Earth, and collect data using the scientific instruments in a lunar mission orbit of 100 km. To enter the lunar mission orbit, four LOI maneuvers will be executed at the planned burn epoch. The first LOI maneuver is the most crucial one for the KPLO be captured in the lunar orbit. The first LOI maneuver provides a highly elliptical orbit around the Moon, and three additional maneuvers are executed to become a circular final lunar orbit. To design the first LOI maneuver, the periselene altitude, inclination, and orbit period of the first captured lunar orbit are considered; in this study, we assume that the captured orbit after the first LOI maneuver will have a periselene altitude of 300 km, inclination of 90°, and orbit period of 12 hr.

During operation, the lunar orbiter will execute the planned first LOI burn at the specific burn epoch. However, a LOI burn can be delayed due to hardware limitations, telemetry delays from the ground system, or other factors. This burn delay leads to different captured lunar orbit after the first LOI maneuver at the delayed LOI burn epoch. As a result, the lunar orbiter orbits around the Moon will have different orbit periods and orbital elements than the planned orbit. In the worst case, the lunar orbiter will significantly stray out of the desired lunar orbit, and more delta-V will be required to recover from the delayed orbits caused by the first LOI burn delay.

For the nominal LOI maneuver for an impulsive burn, the position and velocity vectors of the lunar orbiter after LOI maneuver, $r\left(t_{LOI}^{+}\right)\hspace{0.17em}\text{and}\hspace{0.17em}v\left(t_{LOI}^{+}\right)$, are defined as(1)

where $r\left(t_{LOI}^{-}\right)\hspace{0.17em}\text{and}\hspace{0.17em}v\left(t_{LOI}^{-}\right)$ are the position and velocity vectors before the LOI maneuver, Δv(t_LOI) is the delta-V of the LOI maneuver, and t_LOI is the LOI burn epoch. If the lunar orbiter executes the LOI maneuver beyond t_LOI, the position and velocity vectors of the lunar orbiters are

where $r\left(t_{LOI}^{-}\right)$ and $v\left(t_{LOI}^{-}\right)$ are the position and velocity vectors before the LOI maneuver at the delayed burn epoch, t_{Delay LOI}. In Eq. (2), t_{Delay LOI} = t_LOI + Δt_Delay, so $r\left(t_{Delay\hspace{0.17em}\hspace{0.17em}LOI}^{+}\right)\hspace{0.17em}\ne \hspace{0.17em}r\left(t_{LOI}^{+}\right),\hspace{0.17em}v\left(t_{Delay\hspace{0.17em}LOI}^{+}\right)\ne v\left(t_{LOI}^{+}\right),\hspace{0.17em}r\left(t_{Delay\hspace{0.17em}LOI}^{-}\right)\ne r\left(t_{LOI}^{-}\right)$, and $v\left(t_{Delay\hspace{0.17em}\hspace{0.17em}LOI}^{-}\right)\hspace{0.17em}\ne v\left(t_{LOI}^{-}\right)$. As the delay time interval Δt_Delay increases, the position and velocity vectors after the LOI burn significantly deviate from the nominal values. Fig. 1 shows the lunar orbits with nominal and delayed LOI burns for a finite burn type; the black line presents the nominal case, blue line describes the delayed burn case, and red and purple lines show the LOI burn duration. As shown in Fig. 1, the lunar orbiter has different captured lunar orbits compared to the nominal lunar orbit when the LOI burn does not execute at the exact burn epoch, t_LOI. As a result, the orbital elements and orbit period of the lunar orbiter will change according to the delayed LOI burn.

Fig. 1. Geometry of the nominal and delayed LOI maneuver burns.

Download Original Figure

3 NUMERICAL SIMULATION RESULTS AND ANALYSIS

Numerical simulations are performed to analyze the impacts of a delayed LOI burn. For the simulations, it is assumed that the KPLO adopts a phasing-loop transfer trajectory to the Moon, and a sequence of LOI maneuvers is performed to capture the desired lunar orbit. Numerical simulations are performed using systems tool kit (STK), which is a software package from Analytical Graphics, Inc. (AGI). This tool provides time-dynamic positions and velocities of objects, including satellites and aircraft, and integrated solutions for ground, sea, air, and space can be obtained. Using STK, the trajectory of the KPLO from the Earth to the Moon can be designed for the KPLO program.

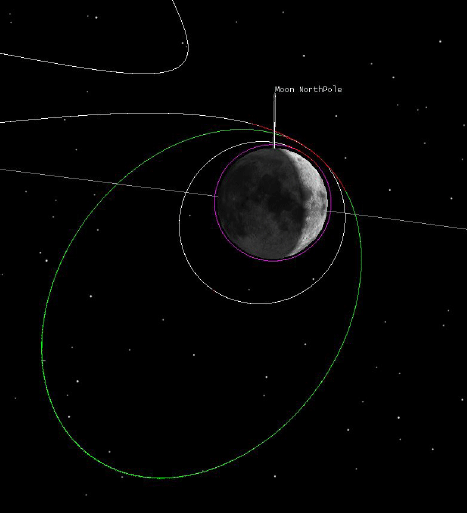
Using STK, targeting parameters can be selected as B-plane parameters, the periselene altitude, inclination, and orbit period of the desired lunar orbit, to generate trajectories of the lunar orbiter. Moreover, control parameters can be defined as orbital elements and delta-V values of the LOI maneuver. With these targeting and control parameters, the mission sequence can be designed. This numerical tool provides simulation results based on defined force modeling, propagation, differential corrector, and engine model. In this study, the finite burn type is selected for the LOI maneuver, and it is assumed that in the propulsion system, the thrust is 120 N and the specific impulse (Isp) is 220 sec. Moreover, the LOI maneuver delta-V values are selected as control parameters, and the periselene altitude, inclination, and orbit period of the first captured lunar orbit are selected as target parameters for the first LOI maneuver. In the nominal case, the nominal captured lunar orbit is obtained, as shown in Fig. 2, by selecting a periselene altitude of 300 km, inclination of 90°, and orbit period of 12 hr; the green line shows the first captured lunar orbit and the white line shows the second captured lunar orbit. Notably, the nominal value of the first LOI maneuver delta-V is 385.1138 m/s and the burn duration is 1,752.273 sec when the KPLO does not have an LOI burn delay.

Fig. 2. Captured lunar orbit after LOI maneuvers (green line: first captured lunar orbit, white line: second captured lunar orbit, purple line: third captured lunar orbit).

Download Original Figure

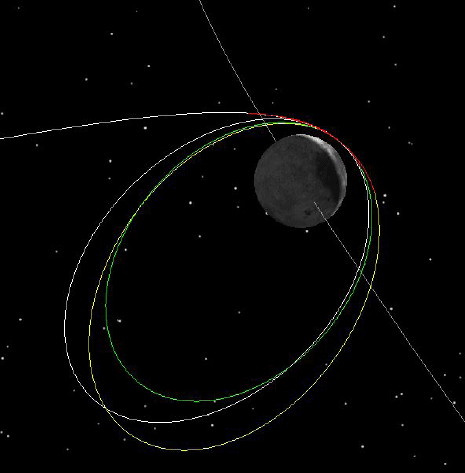
For the delayed LOI burn analysis, the orbital elements, periselene altitude, and orbit period after the first LOI maneuver are analyzed according to the delayed time interval. In addition, the second LOI maneuver delta-V is analyzed to evaluate the consumed fuel with respect to the delayed first LOI burn. Numerical simulation results are analyzed considering the two cases shown in Fig. 3; CASE 1 is a premature LOI burn and CASE 2 is a delayed LOI burn. Premature and delayed LOI burns could occur when the lunar orbiter has hardware limitations, telemetry delays, of onboard clock errors. For each case, it is assumed that the delay time intervals are 10 sec, 100 sec, 3 min, 5 min, and 10 min. Fig. 4 shows the trajectories of the lunar orbiter in the presence of the delayed first LOI burns. As shown in Fig. 4, the lunar orbiter has different lunar orbits according to the delayed time interval; the green colored lunar orbit, which is the nominal first LOI maneuver, has a shorter aposelene altitude compared to the delayed lunar orbits described with white and yellow colors.

Fig. 3. Timing of the two numerical simulation cases compared to the nominal case.

Download Original Figure

Fig. 4. Captured lunar orbit with a delayed LOI burn (green line: nominal first captured lunar orbit, white line: premature first captured lunar orbit, yellow line: delayed first captured lunar orbit).

Download Original Figure

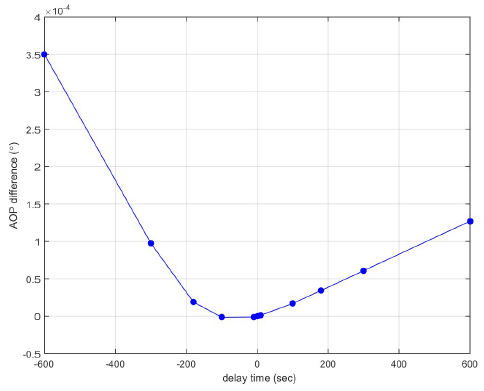
Figs. 5-11 show the differences in orbital elements between nominal and delayed LOI cases for the first LOI maneuver. As shown in Figs. 5 and 6, when the burn delay time increases to 10 min, the differences in the semimajor axis become large more than 1,000 km, and the eccentricity difference increases to 0.05. In Fig. 8, the RAAN difference between the nominal and delayed lunar orbits changes from -6° to 6° when the lunar orbiter executes the first LOI maneuver prior to the planned burn epoch. In contrast, Figs. 7 and 9 show that the inclination and argument of periapsis (AOP) are not significantly changed due to a burn delay. Figs. 10 and 11 present the difference in orbit period and periselene altitude between the nominal and first LOI burn delay cases. The required orbit period of the first captured lunar orbit is 12 hr; however, the resulting orbit period in the presence of the delay burn increases and has a maximum value of 15.5 hr when the delay time interval of the first LOI maneuver grows to 10 min, as shown in Fig. 10. As presented in Fig. 11, the lunar orbiter has a periselene altitude difference of 16 km as the burn delay time increases from 0 to 10 min.

Fig. 5. Difference in the semimajor axis between the desired and delayed lunar orbits.

Download Original Figure

Fig. 6. Difference in the eccentricity between the desired and delayed lunar orbits.

Download Original Figure

Fig. 7. Difference in the inclination between the desired and delayed lunar orbits.

Download Original Figure

Fig. 8. Difference in the RAAN between the desired and delayed lunar orbits.

Download Original Figure

Fig. 9. Difference in the AOP between the desired and delayed lunar orbits.

Download Original Figure

Fig. 10. Difference in the orbit period between the desired and delayed lunar orbits.

Download Original Figure

Fig. 11. Difference in the periselene altitude between the desired and delayed lunar orbits.

Download Original Figure

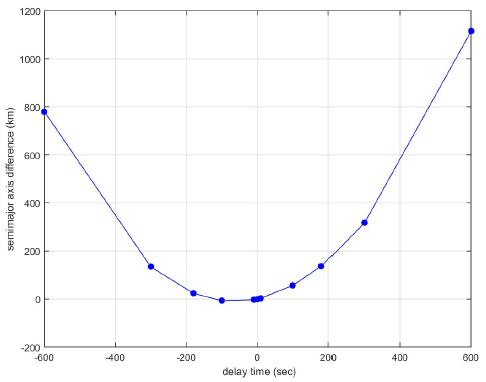
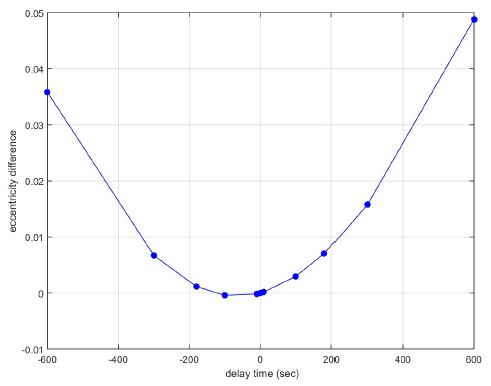
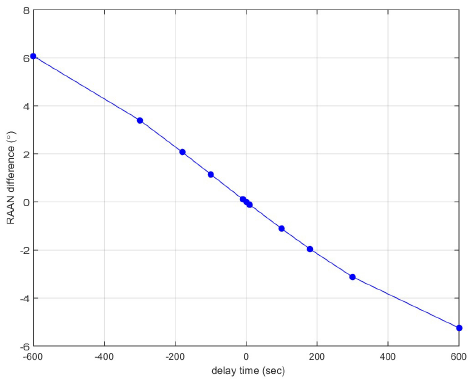
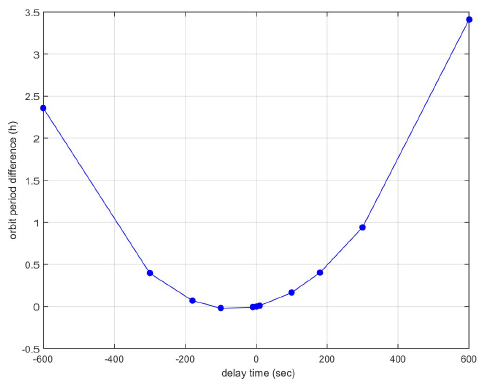
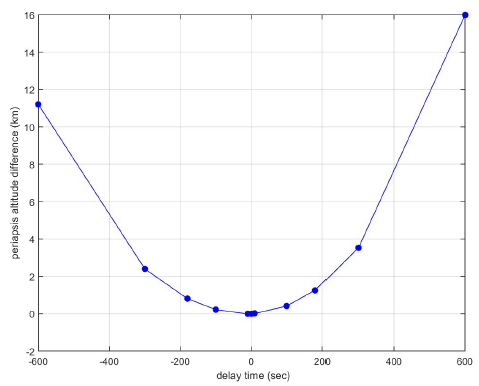
Based on burn epoch, the lunar orbiter shows different simulation results. As shown in Figs. 5-11, the differences in the semimajor axis, eccentricity, RAAN, orbit period, and periselene altitude increase as the delayed burn interval increases to 10 min in both CASE 1 and CASE 2. However, the resulting orbital elements for CASE 1 significantly deviate from the nominal results. For a delayed burn interval of 10 min, the differences in the semimajor axis are 800 km in CASE 1 and 1,100 km in CASE 2, the differences in orbit period are 2.4 hr in CASE 1 and 3.5 hr in CASE 2, and the differences in periselene altitude are 11 km in CASE 1 and 16 km in CASE 2, respectively. From these results, it is clear that the delayed burn case (CASE 2) is more critical than the premature burn case (CASE 1).

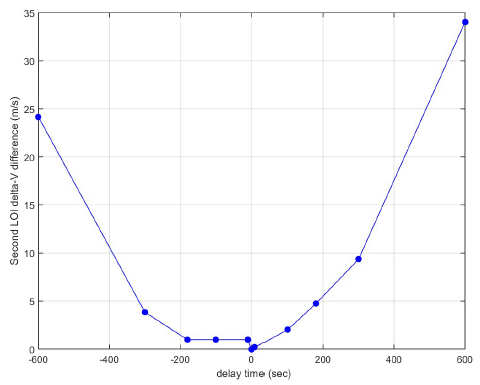
Fig. 12 shows the difference in the second LOI maneuver delta-V according to the first LOI burn delay. The nominal delta-V of the second LOI maneuver is 278.93 m/s; however, as shown, the lunar orbiter requires more delta-V to satisfy the desired second captured lunar orbit, which has an orbit period of 3.5 hr. When the burn delay interval for the first LOI maneuver reaches 10 min, more than 34 m/s of additional delta-V for the second maneuver is required for insertion in the desired second captured lunar orbit, which results in the lunar orbiter consuming more fuel compared to the nominal operation case. If the lunar orbiter has enough of a fuel margin during the operation, this result is not critical and will compensate for the burn delay. If there is not enough fuel, the additional LOI maneuver delta-V will lead to a shortened operational life of the lunar orbiter. As a result, the delay time interval of the maneuver should be considered and reduced during operation to maximize the remaining fuel. In addition, given the desired range of the orbit period for the first captured lunar orbit, and considering the KPLO mission, we propose limits on the delayed burn interval. For example, the burn delay interval should be ≤ 5 min to achieve an orbit period difference of ± 30 min. Furthermore, to maintain a delta-V budget ≤ 5 m/s for the second LOI maneuver, the first burn delay interval should be reduced to within 3 min.

Fig. 12. Difference of 2nd LOI maneuver delta-V between desired and delayed lunar orbits.

Download Original Figure

4 CONCLUSION

This study analyzed the delayed burn for the first LOI maneuver in the KPLO program. The LOI maneuver is the most essential for capturing the lunar orbit because the KPLO will experience a large velocity change during the LOI. Due to hardware limitations, telemetry delays, or other factors during operation, the KPLO may have delayed burn for the LOI maneuver. To analyze the impact of this delayed burn, premature burn and delayed burn cases were considered, with differences from the planned burn epoch of 10 sec, 100 sec, 3 min, 5 min, and 10 min. From numerical simulations, the delayed burn resulted in changes to the orbital elements between planned and delayed captured lunar orbits. The semimajor axis, eccentricity, orbit period, and periselene altitude were significantly affected by a delayed burn for the first LOI maneuver. Moreover, this difference increased significantly as the delayed burn time increased from 0 to 10 min; when the delayed burn time reached 10 min, the differences in the semimajor axis, eccentricity, orbit period, and periselene altitude were larger than 1,000 km, 0.05, 3.4 hr, and 16 km, respectively. In addition, the KPLO will require more delta-V for the second LOI maneuver to compensate for this difference due to the delayed burn in the first LOI maneuver; the additional required delta-V for the second LOI was 34 m/s when the delayed burn interval increased to 10 min. Based on this analysis, the tolerance in the delayed burn interval can be investigated for the KPLO program, and a contingency plan can be prepared for a potential delayed burn for the first LOI maneuver.