1. INTRODUCTION
The F-region ionosphere is highly variable due to a whole number of influences from below (severe meteorological and seismic events) and from above (solar ionizing EUV flux, solar wind conditions, magnetospheric and auroral activities) (e.g., Forbes et al. 2000;Rishbeth & Mendillo 2001;Laštovička 2006;Kim & Hegai 2015;Chung et al. 2016;Kim & Hegai 2016). An ionospheric response to a major earthquake is still far from being fully investigated, although early studies on the subject have been carried out more than 50 years ago when Davies & Baker (1965), Leonard & Barnes (1965), and Row (1966) presented an evidence of perturbations in the F2 layer caused by the M8.4 Alaska Earthquake of March 28, 1964. Row (1967) showed that the ionospheric perturbations could be attributed to the F2-layer electron density of acoustic gravity waves (AGWs) produced by the earthquake in the F-region. A more detailed theoretical treatment of AGWs launched into the ionosphere by seismic shocks was presented by Liu & Yeh (1971) and Yeh & Liu (1974) for the case of an isothermal and dissipationless neutral atmosphere. The data were extrapolated for a realistic neutral atmosphere in a number of studies (e.g., Francis 1973, 1975;Liu & Klostermeyer 1975;Mayr et al. 1984;Maeda 1985;Liang et al. 1998;Sun et al. 2007;Ma 2016). At long distances from an earthquake epicenter, ionospheric perturbations in the F-region due to AGWs of seismic origin are observed as medium- and large-scale traveling ionospheric disturbances (TIDs; Francis 1973, 1975). Seismic TIDs have been registered at distances of more than 3,000 km from epicenters using ionosonde measurements of the F2-layer critical frequency (foF2; Leonard & Barnes 1965;Hegai et al. 2011). The TIDs associated with earthquakes have also been studied using ionospheric total electron content (TEC) data measured by GPS receiver networks (e.g., Calais & Minster 1995;Astafyeva & Afraimovich 2006;Liu et al. 2006;Tsugawa et al. 2011;Cahyadi & Heki 2013).
Another type of ionospheric perturbations detected in the F-region after earthquakes is small amplitude variations in the electron density caused by vertically propagating acoustic waves that were excited by seismic Rayleigh waves traveling along the Earth’s surface with respect to an epicenter. Rayleigh wave signatures in the ionosphere following earthquakes have been measured by Doppler sounding (e.g., Yuen et al. 1969;Tanaka et al. 1984;Artru et al. 2004;Chum et al. 2016) using TEC measurements (e.g., Ducic et al. 2003;Astafyeva et al. 2009;Rolland et al. 2011) and the inspection of ionograms (Maruyama et al. 2012, 2016a, 2016b).
In this work, ionosonde foF2 data are utilized to study perturbations of the peak F2-layer electron density following the M8.8 Chile Earthquake of February 27, 2010, at long distances from its epicenter.
2. DATA ANALYSIS AND DISCUSSION
As reported by the U.S. Geological Survey, the major earthquake with a magnitude of 8.8 and depth of 35 km occurred at 06:34:14 UT on February 27, 2010, at a distance of 335 km from Santiago, the capital of Chile. The epicenter of the earthquake was located at a geographic latitude of 35.9°S and longitude of 72.7°W.
We examine the foF2 values [proportional to (NmF2)1/2] recorded at several ionospheric stations listed in Table 1. The foF2 data were taken from the NGDC SPIDR website (http://spidr.ionosonde.net/spidr/). The index Kp is used to represent the geomagnetic activity around the earthquake. The solar and geomagnetic activities were low in February 2010; the monthly F10.7 and Ap indices were as small as 82.7 and 5, respectively.
Fig. 1 shows the time variation of the observed critical frequency foF2 (a, b, and c) at three ionosonde stations (Hermanus, Madimbo, and Port Stanley) over the period 00:00 to 24:00 UT on February 27, 2010. The average foF2 (<foF2>) of ten quiet days in February 2010, during which the Kp index did not exceed 2 (February 7, 10, 11, 19, 20, 21, 22, 23, 24, and 28, 2010), is superposed to provide reference curves. The vertical lines consequently indicate the moment of the earthquake onset (tEQ), which means the estimated time of arrival of the seismic atmospheric disturbance traveling in the F-region from the epicenter to the ionosonde site along the great circle path (tar). The great circle distances from the epicenter of the earthquake to the selected ionosonde stations are given in Table 1. We estimate the arrival times tar in the simplest way by using the formula tar ~ tEQ + L/V, where L is the value of the great circle distance and V is the speed of the horizontal propagation of the seismic atmospheric disturbance in the F2 layer. The speed of medium- and large-scale seismic atmospheric disturbances, V, is ~800 m/s (e.g., Row 1967;Francis 1973, 1975).
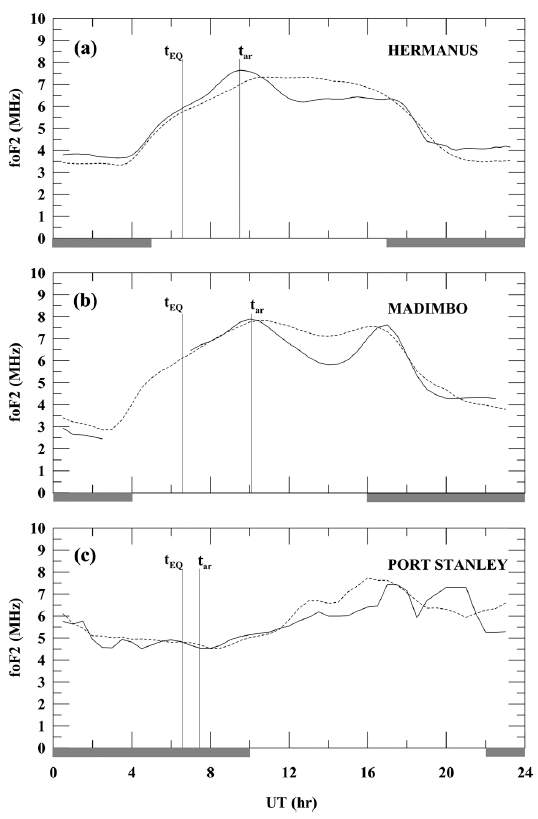
The geomagnetic activity was very low on February 27, 2010, and the Kp index did not exceed 1, while the Kp index did not exceed -3 during the three previous days, which means that the geomagnetic conditions were favorable to identify ionospheric perturbations unrelated to geomagnetic disturbances. After the earthquake, at ~tar, the foF2 at the South African stations Hermanus and Madimbo started to decrease; soon became smaller than <foF2>, by more than 20 %; and recovered to <foF2> in approximately 8 and 5 hr, respectively. Note that the observed depression of foF2 occurred during daytime hours. Fig. 1(c) shows no meaningful negative perturbation in the F2 layer over the South American station Port Stanley at ~tar, although this station is located much closer to the epicenter than the South African stations Hermanus and Madimbo. The other stations listed in Table 1 did also not demonstrate any significant perturbations in foF2 around the estimated time of arrival of large-scale seismic AGWs. It is reasonable to suggest that the negative perturbations in foF2 observed at Hermanus and Madimbo shortly after tar are associated with large-scale long-period AGWs produced in the F2 layer during the Chile Earthquake. The frequency of the predominant seismic AGW near the F2 layer peak at large distance from an earthquake epicenter can be estimated using a simplified theoretical formula (Row 1967; Liu & Yeh 1971; Yeh & Liu 1974), ω ~ ωb(L/hmF2), where is the AGW frequency, ωb is the Brunt–Väisälä frequency (the mean value of ωb/2π in the F2 layer is ~0.001 Hz), and hmF2 is the height of the F2 layer peak at the point of observation. In the case of Hermanus, we obtain ω/2π ~ 3.6×10-5 Hz (for hmF2 taken from the IRI-2016 model: http://omniweb.gsfc. nasa.gov/vitmo/iri2016_vitmo.html/), which means that the AGW period is approximately 7.7 hr. To our knowledge, this is the first evidence of significant perturbation in foF2 produced by seismic AGWs at such a long distance (> 8,000 km) from the epicenter of an earthquake that stipulated the AGWs in the F-region ionosphere. At the same time, the theoretical treatment by Francis (1973) indicates that seismic AGWs with periods between 30 sec and 2 hr are attenuated by a factor of 1/e at a distance of ~5,000 km from the earthquake epicenter. This could explain the absence of any seismic AGW effect on foF2 at other stations located at distances much larger than 5,000 km from the epicenter. Previously, Maruyama et al. (2016) reported a long-distance effect of the Chile Earthquake on the ionospheric F-region based on another mechanism, unrelated to seismic AGWs. They observed short-period (tens of seconds) wavy fluctuations of the virtual height in the daytime F1 region after the earthquake at the European ionospheric station Kazan (L ~ 15,150 km), which were attributed to infrasound waves due to Rayleigh waves excited by the earthquake and propagated to Kazan.
It is important to understand why the nearest station to the earthquake epicenter, Port Stanley (L ~ 2,100 km), did not notably indicate the impact of seismic AGWs on foF2. This could likely be attributed to the fact that the seismic AGWs arrived at Port Stanley at night when the height of the F2 layer peak over Port Stanley was ~320 km, reflecting unfavorable conditions for the seismic AGWs to change the foF2 because a superposition of the seismic AGWs produces perturbation in the ionospheric electron density at long distance, predominantly between altitudes of 180 and 280 km, which is in agreement with Francis (1973).
3. CONCLUSIONS
The present study showed that the M8.8 Chile Earthquake of February 27, 2010, likely produced a long-distance impact on the ionospheric F2 layer at a distance > 8,000 km from the epicenter. Seismic perturbations (reduction) of foF2 have been observed for several hours at the South African stations Hermanus and Madimbo. They exceeded 20 % with respect to quiet time values. We suggest that the mechanism responsible for these perturbations is associated with large-scale AGWs excited in the F-region ionosphere by the earthquake.
